Last Updated on December 29, 2021 at 11:57 am
One of the most common investor mistakes is to assume that the alpha of a fund or stock or portfolio represents the excess return over a market index or relevant benchmark. This is technically and factually wrong and especially important when someone compares funds or stocks based on alpha or any one of standard metrics like Sharpe ratio, Sortino ratio etc. So let us try to understand what does alpha actually represent. First, some facts to justify the title.
Alpha of a fund/stock is NOT excess return above market!
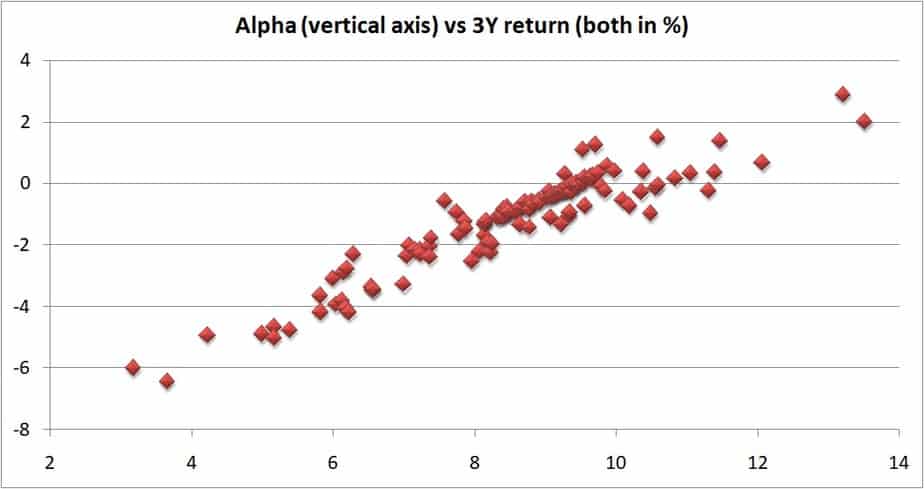
Go to Value Research listing of large-cap funds and download data from the returns tab and risk measures tab. Then list the 3Y return alongside the alpha (calculated for 3Y) and plot the alpha versus the returns and you will get something like this.
What is the big deal! Is not the relationship “roughly” linear? Higher return = higher alpha? Looking at everything “roughly” and talking about it in public like we have understood everything is an infectious disease. I am willing to bet that there will at least be one comment (perhaps only because I said so!) that will claim that this “rough” relationship is all that matters. If you are one such person, please leave now.
Join 32,000+ readers and get free money management solutions delivered to your inbox! Subscribe to get posts via email! (Link takes you to our email sign-up form)
🔥Enjoy massive discounts on our robo-advisory tool & courses! 🔥
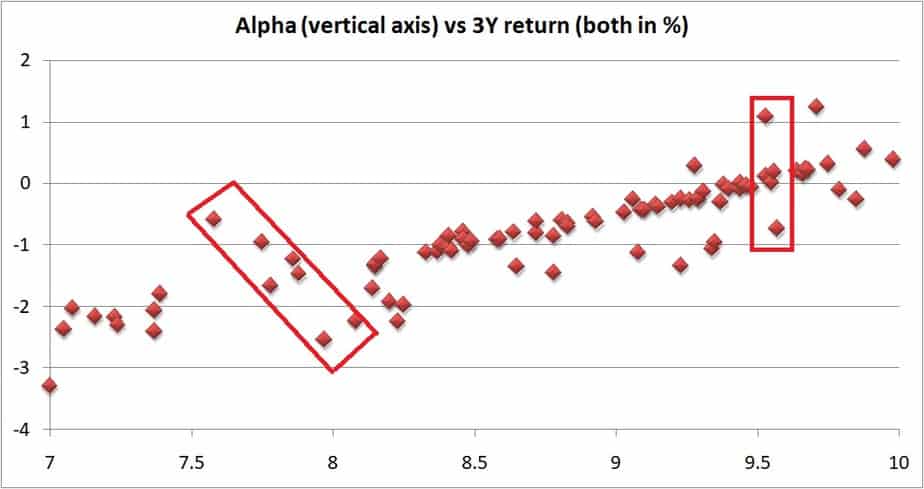
Rigour matters (or is it rigor mortis?). If you agree then look closely.
Notice there are significant pockets where funds with lower return have higher alpha or funds with the same return have a significant spread in alpha. The two funds below have a 1% difference in alpha for pretty much the same return.
This is clear evidence that alpha does not represent excess return.
What is alpha?
Alpha is excess return calculated on a risk-adjusted basis. The job of an active fund manager is to provide excess returns on a risk-adjusted basis, NOT just excess return. Never forget this before comparing an active fund with an index.
Now, you may ask, “why have you not used alpha in your index investing posts?. The reason is that alpha, beta, Sharpe, Sortino etc assume that fund returns fall on a bell curve. This is incorrect. So I prefer downside capture (protection). If you have been thinking alpha = excess return, then you have been using a wrong metric to make a wrong conclusion. Errors almost always compound (& confound)!.
I will reproduce here the discussion on the calculation of alpha from a list of equity mutual funds with low risk and good returns
To calculate alpha, a risk-free return is necessary. According to VR, “Risk-free return is defined as State Bank’s 45-180 days Term Deposit Rate”. So now, let us consider an example. The actual calculation is done with monthly returns and then annualized, but to keep it simple we will consider annual returns.
Let the fund return (over 1Y say) = 10%. Let the risk free return (over same period) = 6%. Let the benchmark return = 8%.
Now funds outperformance over risk free return = 10% – 6% = 4%.
Benchmarks outperformance over risk free return = 8% – 6% = 2%.
We can argue that the actual outperformance of the fund over the risk-free return is 4% – 2% = 2%. Before you say this is needless, I could have just done 10%- 8% = 2%, we need to consider how volatile the fund was wrt to the benchmark. This is calculated by a term known as beta. If beta = 0.8, this means the fund only displayed 80% of the benchmarks volatility. So we write,
The actual outperformance of the fund over the risk-free return =
(Fund return – risk-free return) – (Benchmark return – risk-free return) x beta
That is we reduce the (Benchmark return – risk-free return) by a factor (beta) that represents how identical the funds volatility was wrt benchmark. Let me explain with numbers. So if beta = 0.8
Alpha = (10% – 6%) – (8%-6%) x 0.8
Since the fund was less volatile than the benchmark, we penalize the fund’s outperformance (wrt risk-free return) only by 80% of the benchmarks outperformance (wrt risk-free return). This is known as alpha. It is a measure of excess returns on a risk-adjusted basis. Now suppose the beta was 1.2. This means the fund as 20% more volatile than the benchmark. So the penalty is higher.
While the standard deviation is a measure of absolute risk, beta is a measure of relative risk. It is important to recognise that these quantities assume the presence of a bell-curve like a fund return distribution. This is not true. So take this numbers with a good pinch of salt and treat them as merely indicative. Don’t get married to them.
To summarise, alpha is NOT the excess return of a fund/stock!. It factors in how volatile a fund has been compared to the market. A fund that beats the market but is more volatile than the market in doing so, will have lower alpha than a fund with a lower volatility. Thus even funds with no excess returns can “produce an alpha”.
🔥Enjoy massive discounts on our courses, robo-advisory tool and exclusive investor circle! 🔥& join our community of 7000+ users!
Use our Robo-advisory Tool for a start-to-finish financial plan! ⇐ More than 2,500 investors and advisors use this!
Track your mutual funds and stock investments with this Google Sheet!
We also publish monthly equity mutual funds, debt and hybrid mutual funds, index funds and ETF screeners and momentum, low-volatility stock screeners.
- Do you have a comment about the above article? Reach out to us on Twitter: @freefincal or @pattufreefincal
- Have a question? Subscribe to our newsletter using the form below.
- Hit 'reply' to any email from us! We do not offer personalized investment advice. We can write a detailed article without mentioning your name if you have a generic question.
Join 32,000+ readers and get free money management solutions delivered to your inbox! Subscribe to get posts via email! (Link takes you to our email sign-up form)
About The Author
Dr M. Pattabiraman(PhD) is the founder, managing editor and primary author of freefincal. He is an associate professor at the Indian Institute of Technology, Madras. He has over ten years of experience publishing news analysis, research and financial product development. Connect with him via Twitter(X), Linkedin, or YouTube. Pattabiraman has co-authored three print books: (1) You can be rich too with goal-based investing (CNBC TV18) for DIY investors. (2) Gamechanger for young earners. (3) Chinchu Gets a Superpower! for kids. He has also written seven other free e-books on various money management topics. He is a patron and co-founder of “Fee-only India,” an organisation promoting unbiased, commission-free investment advice.Our flagship course! Learn to manage your portfolio like a pro to achieve your goals regardless of market conditions! ⇐ More than 3,000 investors and advisors are part of our exclusive community! Get clarity on how to plan for your goals and achieve the necessary corpus no matter the market condition is!! Watch the first lecture for free! One-time payment! No recurring fees! Life-long access to videos! Reduce fear, uncertainty and doubt while investing! Learn how to plan for your goals before and after retirement with confidence.
Our new course! Increase your income by getting people to pay for your skills! ⇐ More than 700 salaried employees, entrepreneurs and financial advisors are part of our exclusive community! Learn how to get people to pay for your skills! Whether you are a professional or small business owner who wants more clients via online visibility or a salaried person wanting a side income or passive income, we will show you how to achieve this by showcasing your skills and building a community that trusts and pays you! (watch 1st lecture for free). One-time payment! No recurring fees! Life-long access to videos!
Our new book for kids: “Chinchu Gets a Superpower!” is now available!
Must-read book even for adults! This is something that every parent should teach their kids right from their young age. The importance of money management and decision making based on their wants and needs. Very nicely written in simple terms. - Arun.Buy the book: Chinchu gets a superpower for your child!
How to profit from content writing: Our new ebook is for those interested in getting side income via content writing. It is available at a 50% discount for Rs. 500 only!
Do you want to check if the market is overvalued or undervalued? Use our market valuation tool (it will work with any index!), or get the Tactical Buy/Sell timing tool!
We publish monthly mutual fund screeners and momentum, low-volatility stock screeners.
About freefincal & its content policy. Freefincal is a News Media Organization dedicated to providing original analysis, reports, reviews and insights on mutual funds, stocks, investing, retirement and personal finance developments. We do so without conflict of interest and bias. Follow us on Google News. Freefincal serves more than three million readers a year (5 million page views) with articles based only on factual information and detailed analysis by its authors. All statements made will be verified with credible and knowledgeable sources before publication. Freefincal does not publish paid articles, promotions, PR, satire or opinions without data. All opinions will be inferences backed by verifiable, reproducible evidence/data. Contact information: letters {at} freefincal {dot} com (sponsored posts or paid collaborations will not be entertained)
Connect with us on social media
- Twitter @freefincal
- Subscribe to our YouTube Videos
- Posts feed via Feedburner.
Our publications
You Can Be Rich Too with Goal-Based Investing
Published by CNBC TV18, this book is meant to help you ask the right questions and seek the correct answers, and since it comes with nine online calculators, you can also create custom solutions for your lifestyle! Get it now.Gamechanger: Forget Startups, Join Corporate & Still Live the Rich Life You Want
This book is meant for young earners to get their basics right from day one! It will also help you travel to exotic places at a low cost! Get it or gift it to a young earner.Your Ultimate Guide to Travel
This is an in-depth dive into vacation planning, finding cheap flights, budget accommodation, what to do when travelling, and how travelling slowly is better financially and psychologically, with links to the web pages and hand-holding at every step. Get the pdf for Rs 300 (instant download)